
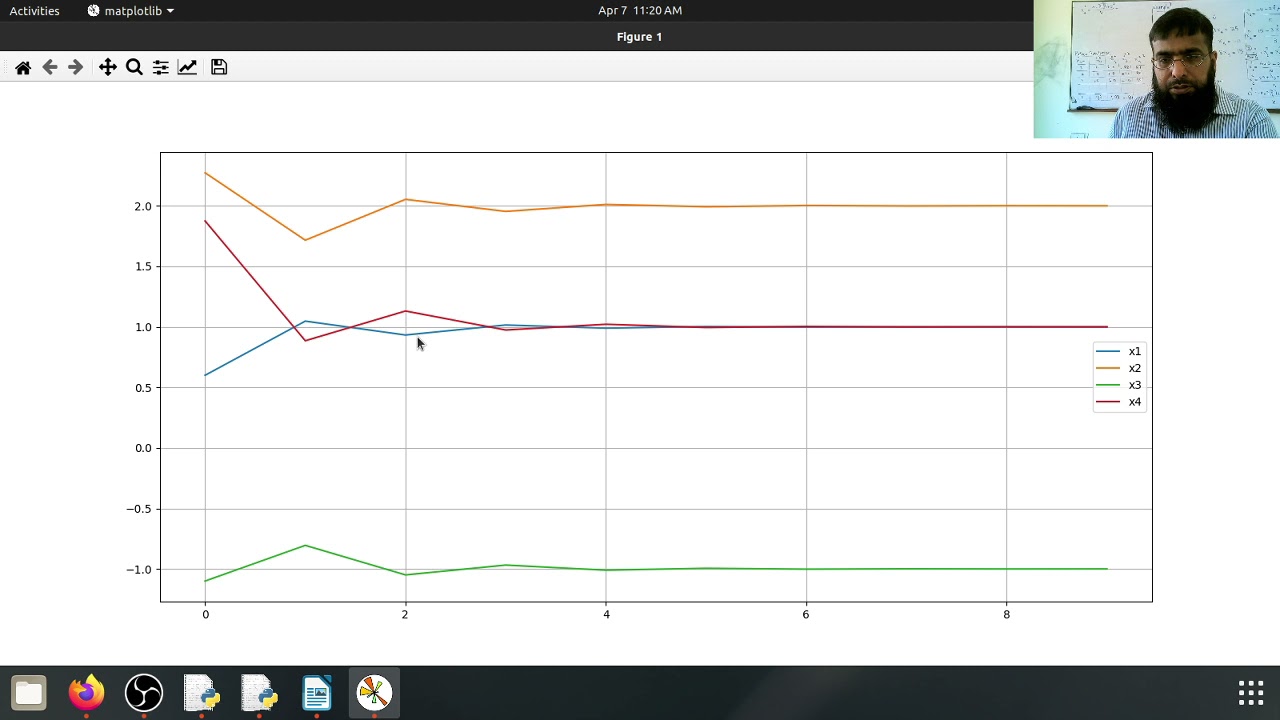
The G-S use the updated values computed in the loop just before and take twice less time to solve the problem than Jacobi (Jacobi don't use updated values). Is there a Python 2.7 package that contains a Gauss-Siedel solver for systems with more than 3 linear algebraic equations containing more than 3 unknowns A simple example of the sort of problem I would like to solve is given below. It works using loop but loops are slow (~1s per iteration), so I tried to vectorize the expression and now the G-S (thus SOR) don't work anymore. I did the Jacobi, Gauss-seidel and the SOR using Numpy. #LnormInf corresponds to the absolute value of the greatest element of the vector.I wrote a code to solve a heat transfer equation (Laplace) with an iterative method. I want to make proram for Gauss Seidel implementation And I want to save every iteration on one JSON But I have some trouble because my code only save the last iteration to Json import json iteras. Print ("The solution vector in iteration", iter1, "is:", x)
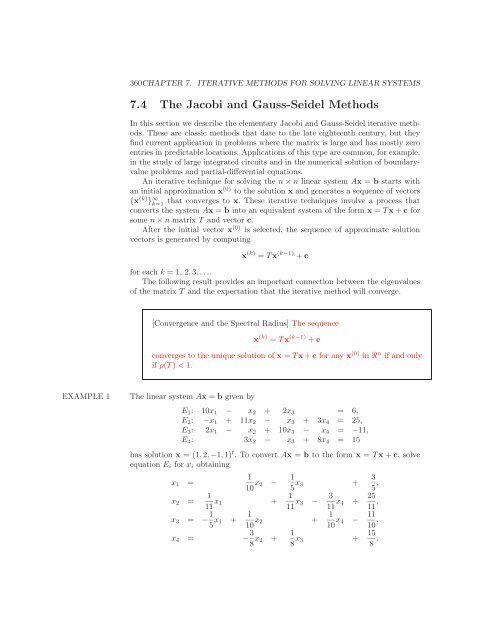
H is an iteration matrix that depends on A and B. Where x k + 1 and x k are approximations for the exact root of Ax B at (k + 1)th and kth iterations. Gauss-Seidel Method Python The Gauss-Seidel method is an iterative method for solving a linear system of equations, typically represented by the matrix equation Ax b, where A is a matrix of coefficients, x is a vector of unknowns, and b is a vector of constants. The general iterative formulas can be given as: x k + 1 Hx k k 1, 2, 3. As the Gauss-Seidel algorithm above involves a triple-loop (the iterative while-construct, pluss one loop in each physical direction), the naive python implementation above must be expected to be computationally expensive.
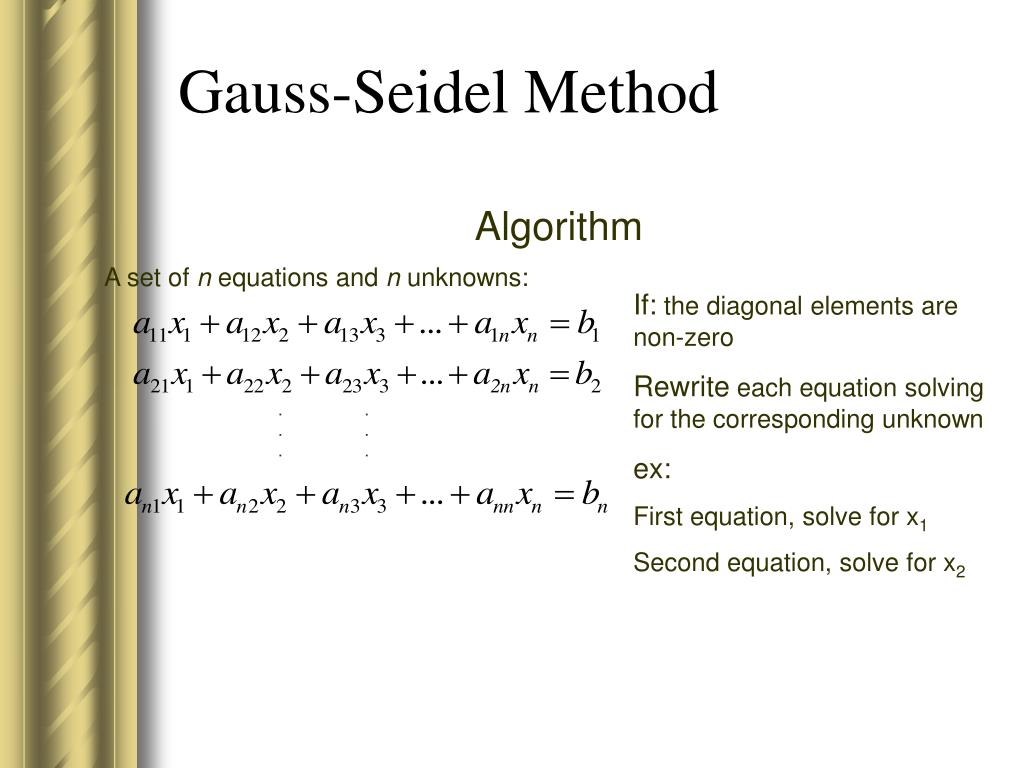
Formula: The formula to find the Gauss Seidel Method is given as: x (k+1) L-1(b-Uxk) Where. def gauss_seidel(A, b, tolerance, max_iterations, x): Iterative methods Jacobi and Gauss-Seidel in numerical analysis are based on the idea of successive approximations. Gauss Seidel iteration method is also known as the Liebmann method or the method of successive displacement which is an iterative method used to solve a system of linear equations. Instead I created my own little function that with the help of a permutation matrix as seen in another answer of mine permutation matrix will produce the solution (x vector) for any square matrix, including those with zeros on the diagonal. The function should also return the values of 1, and Frobenius norms of.

I know this is old but, I haven't found any pre existing library in python for gauss - seidel. Write a python function to generate Gauss Seidel iteration for a given square matrix.


 0 kommentar(er)
0 kommentar(er)
